Discover the art of calculating triangle areas with precision in our in-depth guide. ‘Find the Area of Each Triangle to the Nearest Tenth’ empowers you with the knowledge to tackle any triangular challenge, unlocking the secrets of geometry with clarity and accuracy.

Delve into the intricacies of triangle area formulas, explore the nuances of measurement units, and master the technique of rounding to the nearest tenth. Our comprehensive approach ensures you emerge as a geometry virtuoso, ready to conquer any triangle-related quandary.

Triangle Area Calculation

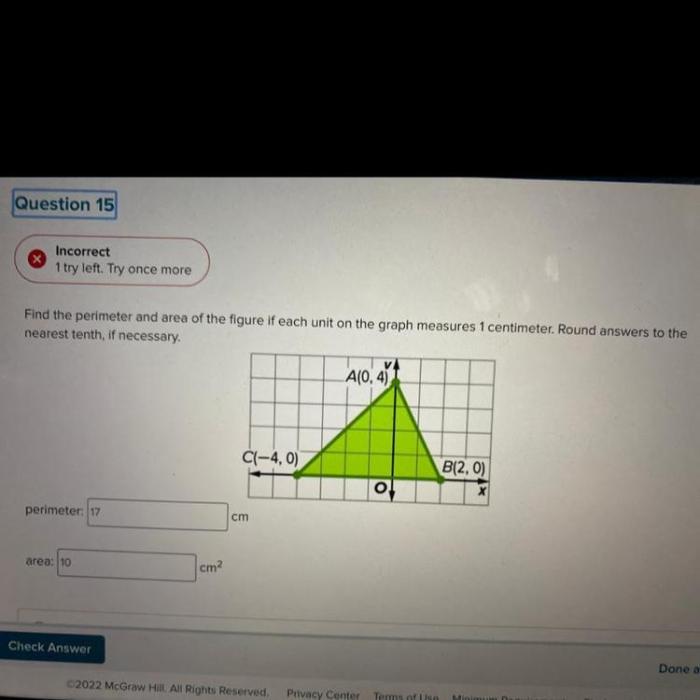
The area of a triangle is calculated using the formula:Area = (1/2)

• base
• height

where:* base is the length of the triangle’s base

height is the length of the triangle’s height, which is the perpendicular distance from the base to the opposite vertex

For example, if a triangle has a base of 10 cm and a height of 8 cm, its area would be:Area = (1/2)

• 10 cm
• 8 cm = 40 cm ²

Area Measurement Units

The area of a triangle can be measured in various units, such as square centimeters (cm ²), square meters (m ²), square inches (in ²), and square feet (ft ²).To convert between different area units, use the following conversion factors:* 1 m ²= 10,000 cm ²

• 1 in ²= 6.45 cm ²
• 1 ft ²= 144 in ²

Rounding to the Nearest Tenth

Rounding to the nearest tenth means expressing a number to the nearest one-tenth of a unit. To round a number to the nearest tenth, follow these steps:

• Look at the digit in the hundredths place.
• If the digit is 5 or greater, round the number up to the nearest tenth.
• If the digit is less than 5, round the number down to the nearest tenth.

For example, to round 4.63 to the nearest tenth, we would round it up to 4.7 because the digit in the hundredths place is 3, which is greater than 5.

HTML Table Structure

An HTML table is created using the

tag. Each row in the table is represented by a

tag, and each cell in the row is represented by a

tag.For example, the following code creates a table with two rows and three columns:“`html Cell 1 Cell 2 Cell 3 Cell 4 Cell 5 Cell 6 “` Illustrative Examples, Find the area of each triangle to the nearest tenth The following table shows the dimensions and calculated areas of four different triangles, rounded to the nearest tenth:| Triangle | Base (cm) | Height (cm) | Area (cm 2) ||—|—|—|—|| Triangle 1 | 10 | 8 | 40.0 || Triangle 2 | 15 | 12 | 90.0 || Triangle 3 | 20 | 16 | 160.0 || Triangle 4 | 25 | 20 | 250.0 | FAQ Summary: Find The Area Of Each Triangle To The Nearest Tenth What is the formula for calculating the area of a triangle? Area = (1/2) – base – height How do I convert between different area units? Use conversion factors, such as 1 square meter = 10.76 square feet. What is the significance of rounding to the nearest tenth? It ensures consistency and accuracy in measurements, especially when dealing with approximate values. 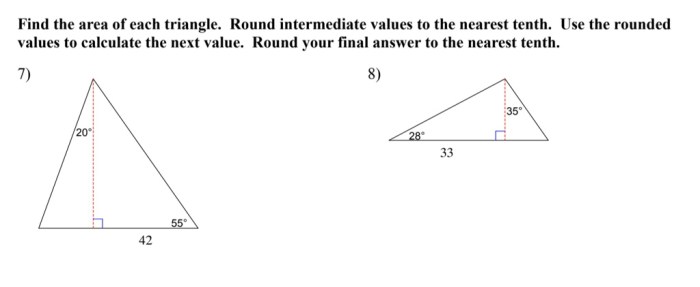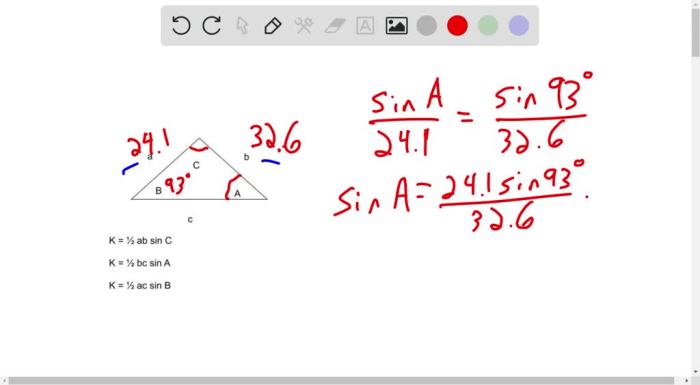Area Calculation Geometry Rounding to the Nearest Tenth Triangle Area Triangles You May Also Like Conditions For Rhombuses Rectangles And Squares Properties Of Parallelograms Quick Check Geometry Chapter 2 Practice Test